Separation Theory
by Ivanka Barzashka and Ivan Oelrich
The following discussion ignores the internal mechanism of how a centrifuge works and treats the machine as a black box. To understand the separative properties of a centrifuge, only material flows in and out of the machine will be considered. Assuming that no material is created or lost, we can track the flow of mass and describe the degree to which the concentration of the desired isotope is increased.
For any separating element, a stream of material of certain concentration enters and two streams of material emerge – one with higher and the other with lower concentration of the desired isotope than the input. The input material is called the feed. The output stream with high concentration is called the product (or heads) and the stream with low concentration – the waste (or tails). The general principles that describe the isotope separation process are the same for any single separating element or multiple elements linked together to function as a single unit, called a cascade. A detailed discussion of cascade theory is available in the section on Cascade Theory.
Input Material for a Gas Centrifuge
The material that enters and leaves a gas centrifuge is uranium hexafluoride (commonly referred to as hex or UF6), a gaseous compound of uranium. The uranium in UF6 is a mixture of primarily two isotopes, U-238 and U-235. Fortuitously, the fluorine has only one isotope, so any mass difference between molecules of UF6 is due to the uranium. For uranium enrichment, the isotopic composition of interest is the amount of U-235 present with respect to the total mass of the substance, mostly U-238. Natural uranium contains a third isotope, U-234, but typically in concentrations of 50 to 60 parts per million. U-234 is typically ignored in mathematical discussions of enrichment and uranium is considered simply as a two component system. (U-234 concentrations in natural uranium vary slightly from mine to mine and these small differences in U-234 concentration can help identify the source of illicit nuclear material. Such tracking is one example of nuclear forensics.)
In a gas centrifuge used for uranium enrichment, the feed is typically natural uranium with a composition of 0.711 percent U-235. In a batch recycling process, it is possible to use low-enriched uranium (LEU), containing 3.0 to 5.0 percent U-235 as feed to produce bomb-grade uranium.
When flow rates or total amounts are reported, care should be taken to note whether the quantities are measured in terms of uranium mass or UF6 mass. The mass of uranium hexafluoride (UF6) is equal to 1 uranium atom and 6 fluoride atoms. The molecular weight is therefore given by: 
This makes the mass of uranium approximately 67.6 percent of that of uranium hexafluoride. IAEA reports usually describe material feed rates in terms of UF6. However, flow rates should be converted to uranium when calculating separative properties such as the separative power.
Defining Physical Concepts
Before describing how to quantify the separation capability of a centrifuge, several physical terms must be defined.
The relative concentration of the isotope that is being enriched is called the isotopic composition and measured as a fraction or percent of the total mass. The isotopic composition of the feed is xf . Neglecting the U-234, the concentration of the U-238 in the feed will be 1-xf .
The feed goes into the separating unit at a flow rate or throughput of F. For an individual machine, throughput is usually measured in grams or kilograms per hour and for a cascade in tons per year.
The product stream has concentration xp and flow rate P. The waste has a concentration and flow rate of xw and W.
The cut of the separating element is defined by the ratio between the product and feed flow:
Since no material is lost in the separation process, the amount of U-235 coming into the separating unit as feed should equal the amount of U-235 coming out as product and waste. The material balance equation relates the compositions and flow rates of the feed, product and waste.
In terms of the cut, the material balance equation yields:
The relative isotopic abundance R is another parameter that describes the composition of the material. It relates the quantity of the two isotopes in the mixture by taking the ratio of the desired isotope (U-235) to the undesired one (U-238). For example, the relative isotopic abundance of the waste is given by:
Separation Factor
The separation factor (also called single stage separation factor or simple process factor) measures the degree of separation achieved by a separation element. It is defined as the ratio between the relative isotopic abundance of the product and that of the waste.
Because the separation factor is often close to unity, the separation gain ε (also called the simple process difference) is sometimes useful.
For separation through gaseous diffusion, each discrete separative element enriches the material only slightly and the separation factor is close to one. When single units are interconnected in a cascade, the overall separation factor of the series of machines is the product of all the individual units’ separation factors multiplied together.
In general, there is a tradeoff between separation factor and throughput: during a fixed time, a machine can enrich a large amount of material to a small degree or a small amount of material to a large degree. The greatest separation occurs at total reflux — i.e. letting the centrifuge run with a fixed charge of UF6 but without adding or removing any material. Clearly, this does not produce any separated product. At the opposite extreme, quickly running large quantities of UF6 through the machine produces a lot of product, but the centrifuge does not have time to work on the mixture to achieve separation. The objective in operating a separative device is to balance the separation and throughput to maximize the overall performance.
Separative Work and Separative Power
We need a way to define and measure the overall effect and capacity of a centrifuge or other enriching element. For that purpose, a separative work and a separative power have been defined. The separative work refers to a certain enhancement of enrichment on a certain amount of material. The separative power describes the rate at which the separative work is done on that material.
The degree of enrichment produced on a mass of material by any particular machine depends on the composition of the feed. No enrichment takes place, obviously, if a centrifuge is working on an isotopically-pure gas, even though the machine is operating exactly in the same way as it would if it were filled with a gas made of two isotopes. A measure of effectiveness of the machine should, therefore, be independent of the composition of the feed material and is more complex than simply the degree of enrichment produced by a machine with a particular feed material.
Paul Dirac first treated this problem in 1941 and developed the notion of the value of an enriched gas, which closely correlates to entropy. The performance of the enriching device is then defined in terms of net value added to a given amount of the material that passed through the machine for enrichment. The value of a gas is simply its mass times a value function V(x). The separative work δU of a centrifuge is the net gain in value, ∆V, which is the value of the output (the value of the product plus the value of the waste) minus the value of the input (feed material).
The value function can be derived either from the change in entropy for the gas [3] or from the general equation for separative work (above).The latter mathematically defines the value function so that the separative work of an enrichment device is independent of concentration. The value function can be graphed on a simple plot or can easily be calculated for a given concentration of material. The equation for the value function is:
where x is the concentration of the material, which can be that of either the feed, product or waste. (Actually, in the derivation, the concentrations of the feed, product, and waste come into the equation, but under the close-approximation condition, it can be assumed they are all close enough to be considered equal.)
Note that the value is a dimensionless number and a thermodynamic intensive quantity. Increasing the value by 1 is called a separative work unit, or SWU. Separative work is measured in units of kg∙SWU, which is the increase of value by 1 of a kilogram of material. Since almost all references to separative work units are kg∙SWUs, the kg is sometimes dropped and the unit is called, simply, a SWU. Very early texts from Britain and the United States will sometimes refer to pound∙SWUs and the separative work of an entire enrichment plant will be described in units of ton-SWUs, so it is best to always include the mass unit when using SWUs and explicitly refer to kg∙SWUs. Note that the numeric result for the value of any given material is not really important, what measures the effectiveness of the centrifuge is the net change in value. It turns out that using the value function equation above, the value of an equal mixture of isotopes is zero.
Following the analogy between work and power with power being work per unit time, the separative power is simply the separative work done in a fixed time. Separative power is usually expressed in units of kg∙SWU/hr for individual machines and ton∙SWU/year for entire enrichment factories.
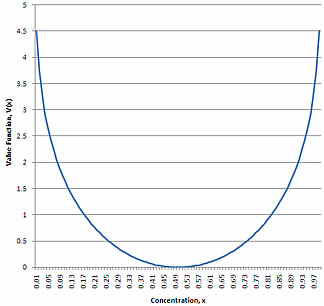The value as a function of concentration is shown in the figure adjacent. Note that the separative work required for any fixed, or even relative, increase in concentration depends on the concentration, as was hypothesized above. So a 10 percent increase from 0.10 to 0.11 U-235 concentration takes more separative work than the 10 percent increase going from 0.50 to 0.55. (Beyond a concentration of 0.50, concentration of U-235 becomes increasingly difficult because U-235 becomes the majority isotope and U-235 and U-238 simply trade places mathematically.)
Knowing the value added by a given machine, one can quickly see how much enrichment occurs at any given feed concentration. Conversely, one can calculate the amount of value that must be added to achieve a certain enrichment of a given amount of material and then calculate how much centrifuge work would be required to produce that increased value. Enrichment value allows a direct comparison of the amount of separative work required to produce, for example, a ton of 5 percent U-235 for a nuclear reactor and 50 kg of 95 percent U-235 for a nuclear bomb.
Examples
We can calculate the amount of separative work needed to produce enough LEU to power a pressurized water reactor (PWR) with a 1000 MWe (megawatt electric) gross capacity, which is the electric power that the reactor produces. Factoring in the inefficiency of the PWR, gives a thermal capacity (or the energy that needs to be produced in fission) of 3250 MWt (megawatt thermal). An average reactor has a capacity factor of 0.8, which means that it produced about 80 percent of the maximum net electricity that it could have generated at constant full-capacity operation. Current U.S. reactors have an average capacity of about 90 percent.
Although most of the energy in the reactor is produced from fission of U-235, there is some energy produced due to internal breeding. An accurate calculation of the amount of fuel needed to power a reactor requires the use of a computer program. According to one source, a reactor with the above characteristics with a burnup of 33,000 MWd/MT (megawatt days per ton of fuel) and three zone fueling requires about 27,300 kg uranium containing 3.3 percent U-235 [2]. Not all of the U-235 is burned in the reactor. There is less than 1 percent present when the fuel rods are removed from the reactor core.
If fuel is not recycled, factoring in a 1 percent loss during conversion and fuel fabrication, a capacity of 109 t SWU is needed to produce 27,500 kg LEU. This is valid assuming we are enriching natural uranium starting at a concentration of 0.711 percent and enriching to 3.3 percent LEU with tails of 0.3 percent.
To enrich an IAEA “significant quantity” of HEU (equivalent to 25 kg U-235 or 27.8 kg HEU at 90 percent concentration) from natural uranium with a tails of 0.3 percent requires 5360 kg SWU. This means that if we use the separative capacity of a plant sized to produce enough LEU for a reactor, we could produce 565 kg of HEU or enough for 20 nuclear bombs. In this scenario, we would be throwing away 42 percent of the U-235 present in the feed.
If fuel grade uranium (LEU at 3.3 percent) is further enriched to get HEU and the tails are set at 0.3 percent, the separative capacity of the enrichment plant would allow for the production of enough material for 52 bombs in one year. About 7.5 percent of the U-235 present in the feed (in this case LEU) will be discarded in the process. If the same scenario is used, but with a tails of 1.2 percent, 30 percent of the U-235 present in the feed will be discarded, but the HEU produced in a year will be enough for 85 bombs. Note that in these scenarios, LEU is not the limiting quantity.
References
[1] Olander, D. R. (1972). Technical Basis of the Gas Centrifuge. Advances in Nuclear Science and Technology, 6, 105-165.
[2] Pigford, T. H., & Levi, H. W. (1981). Fuel Cycles for Nuclear Reactors. In M. Benedict (Author), Nuclear chemical engineering (2nd ed., pp. 84-156). New York: McGraw-Hill.
[3] Whitley, S. (1984). Review of the gas centrifuge until 1962. Part I: Principles of separation physics. Reviews of Modern Physics, 56(1), 41-66.
